Assignments Class 11 Mathematics Binomial Theorem

Please refer to Assignments Class 11 Mathematics Binomial Theorem Chapter 8 with solved questions and answers. We have provided Class 11 Mathematics Assignments for all chapters on our website. These problems and solutions for Chapter 8 Binomial Theorem Class 11 Mathematics have been prepared as per the latest syllabus and books issued for the current academic year. Learn these solved important questions to get more marks in your class tests and examinations.

Binomial Theorem Assignments Class 11 Mathematics

Question. What is the coefficient of x³ in (3 – 2x)/(1 + 3x) ?
(a) – 272
(b) – 540
(c) – 870
(d) – 918 

Question. The value of ⁵⁰C₄ + ⁶∑_r=1 ^56–rC₃
(a) ⁵⁵C₄
(b) ⁵⁵C₃
(c) ⁵⁶C₃
(d) ⁵⁶C₄   

Question. The coefficient of x³ in the expansion of (x –1/x)⁷ is :
(a) 14
(b) 21
(c) 28
(d) 35 

Question. The formula (a + b)^m  = a^m + ma^m–1b + m(m – 1)/1.2 a^m–2b² +…….. holds when
(a) b < a
(b) a < b
(c) | a | < | b |
(d) | b | < | a | 

Question. Match the terms given in column-I with the terms given in column-II and choose the correct option from the codes given below. 

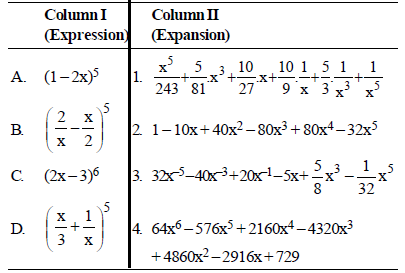
Codes
     A B C D
(a) 2 4 3 1
(b) 2 3 4 1
(c) 1 3 4 2
(d) 1 4 3 2

Question. Number of terms in the expansion of (1+ 5√2x)⁹ + (1– 5√2x)⁹ is
(a) 2
(b) 3
(c) 4
(d) 5 

Question. If the rth term in the expansion of (x/3 – 2/x²)¹⁰ contains x⁴, then r is equal to
(a) 2
(b) 3
(c) 4
(d) 5

Question. A positive value of m for which the coefficient of x² in the expansion (1 + x)^m is 6, is
(a) 3
(b) 4
(c) 0
(d) None of these 

Question. If x is positive, the first negative term in the expansion of (1+ x)^27/5 is
(a) 6th term
(b) 7th term
(c) 5th term
(d) 8th term 

Question. If x⁴ occurs in the rth term in the expansion of (x⁴ + 1/x³)¹⁵ , then what is the value of r ?
(a) 4
(b) 8
(c) 9
(d) 10 

Question. The sum of the series ²⁰C₀ – ²⁰C₁ +²⁰C₂ – ²⁰C₃+ …..–…..+ ²⁰C₁₀ is
(a) 0
(b) ²⁰C₁₀
(c) ^–20C₁₀
(d) 1/2 ²⁰C₁₀ 

Question. The middle term in the expansion of (1+1/x²)(1+ x²)ⁿ is
(a) ²ⁿC_n x²ⁿ
(b) ²ⁿC_n x^–2n
(c) ²ⁿC_n
(d) ²ⁿC_n–1 

Question. The coefficient of x^p and x^q (p and q are positive integers) in the expansion of (1 + x)^{p + q} are
(a) equal
(b) equal with opposite signs
(c) reciprocal of each other
(d) None of these 

Question. If x4 occurs in the tth term in the expansion of (x⁴ + 1/x³)¹⁵ , then the value of t is equal to :
(a) 7
(b) 8
(c) 9
(d) 10 

Question. If 7⁹ + 9⁷ is divided by 64 then the remainder is
(a) 0
(b) 1
(c) 2
(d) 63 

Question. What is the middle term in the expansion of (x√y/3 – 3/y√x)
(a) C(12, 7) x³ y^–3
(b) C(12, 6)x^–3 y³
(c) C(12, 7) x^–3 y³
(d) C(12, 6) x³ y^–3 

Question. The total number of terms in the expansion of (x + a)⁵¹ – (x – a)⁵¹ after simplification is
(a) 102
(b) 25
(c) 26
(d) None of these 

Question. What is the coefficient of x³ y⁴ in (2x + 3y²)⁵ ?
(a) 240
(b) 360
(c) 720
(d) 1080 

Question. How many terms are present in the expansion of (x² + 2/x²)¹¹ ?
(a) 11
(b) 12
(c) 10
(d) 11! 

Question. The middle term in the expansion of (1+1/x²)ⁿ (1+ x²) is
(a) ²ⁿC_n x²ⁿ
(b) ²ⁿC_n x^–2n
(c) ²ⁿC_n
(d) ²ⁿC_n–1   

Question. Find the largest coefficient in the expansion of (4 + 3x)²⁵. 

Question. The number of zero terms in the expansion of (1+ 3√2x)⁹ + (1– 3√2x)⁹ is
(a) 2
(b) 3
(c) 4
(d) 5 

Question. In the expansion of (1 + x)⁵⁰, the sum of the coefficients of odd powers of x is :
(a) 0
(b) 2⁴⁹
(c) 2⁵⁰
(d) 2⁵¹

Question. If ‘n’ is positive integer and three consecutive coefficient in the expansion of (1 + x)ⁿ are in the ratio 6 : 33 : 110, then n is equal to :
(a) 9
(b) 6
(c) 12
(d) 16 

ASSERTION – REASON TYPE QUESTIONS

(a) Assertion is correct, reason is correct; reason is a correct explanation for assertion.
(b) Assertion is correct, reason is correct; reason is not a correct explanation for assertion
(c) Assertion is correct, reason is incorrect
(d) Assertion is incorrect, reason is correct. 

Question.

Question. Assertion. In the binomial expansion (a + b)ⁿ, r^th term is ⁿC_r a^{n – r}.b^r.
Reason. If n is odd, then there are two middle terms. 

Question. Assertion : The term independent of x in the expansion of (x + 1/x + 2)^m is (4m !)/)2m!)².
Reason : The coefficient of x6 in the expansion of (1 + x)ⁿ is ⁿC₆.

Question. Assertion: Number of terms in the expansion of [(3x + y)⁸ – (3x – y)⁸] is 4.
Reason: If n is even, then {(x + a)ⁿ – (x – a)ⁿ} has n/2 terms. 

Question. Assertion : The rth term from the end in the expansion of (x + a)ⁿ is ⁿC_{n – r + 1} x^{r – 1} a^{n –r + 1}.
Reason : The rth term from the end in the expansion of (x + a)ⁿ is (n – r + 2)^th term.  

Question. Assertion : ⁿC₀ + ⁿC₂ + ⁿC₄ + ….. = 2^{n – 1}
Reason : ⁿC₁ + ⁿC₃ + ⁿC₅ + …… = 2^{n – 1}